![]() (1)
(1)
Determination of Avogadro's Number
MOTIVATION:
"It is impossible, perhaps, to speak on this point without committing oneself beyond what present facts will sustain; and yet it is equally impossible, and perhaps would be impolitic, not to reason upon the subject. Although we know nothing of what an atom is, yet we cannot resist forming some idea of a small particle, which represents it to mind; and though we are in equal, if not greater ignorance of electricity .... yet there is an immensity of facts which justify us in believing that the atoms of matter are in some way endowed or associated with electrical powers, to which they owe their most striking qualities and amongst them their mutual chemical affinity."
FARADAY (on the electrical properties of atoms)
In the study of physics we encounter numerous "fundamental constants." Among these are the universal gravitational constant, G; the speed of light in vacuum, c; Planck's constant of action, h; Avogadro's number, No; and the elementary charge, e. How does one measure the speed of light when it travels so fast that it circles the globe five times in one second? How does one measure the force of attraction ( ~10-10 N) between two one-kilogram masses suspended one meter apart? Where do you start when counting the number of atoms ( ~1023) in a gram-molecular mass of some material?
Interestingly, the experiments which answer these questions are always conceptually very simple -- well within the capability of any competent student if high precision is not required. A student begins to approach maturity in physics when he/she forgets that G = 6.67 x 10-11 N m2 / kg2, and begins to wonder, "How do we know?" and "What does it mean?" The exercise which follows illustrates how one approaches such questions. Strive for understanding -- not just rote following of instructions.
SPECIFIC OBJECTIVES:
When you have completed this experimental activity, you should be able to: (1) define and give appropriate units for elementary charge, Avogadro's number, gram-molecular mass, ion, electrolysis, and valence; (2) conduct an experiment to determine the mass of a single copper atom and the value of Avogadro's number.
THEORY:
Evidence around 1900 indicated rather conclusively that both matter and electricity were "atomic"; that is to say they appear in experiments in units of finite and restricted size. The smallest positive charge a body can acquire is that of an object which has lost one of its electrons. We still refer to this charge as +e. Correspondingly, if a body acquires N extra electrons, it will have a negative charge which we can represent as -Ne.
The value of e was first accurately determined in 1909 by Robert Millikan when he measured the excess charge on extremely small drops of oil. In that classic experiment, the value of the electronic charge was found to be -1.602 x 10-19 coulomb.
Earlier in the 19th century, chemists had shown that one gram atomic mass of any element contains the same number (No) of atoms. This number, called Avogadro's number, will be found in this investigation using the above knowledge of the electronic charge. The apparatus used will involve passing an electric current between copper electrodes immersed in a solution of copper sulfate (CuSO4).
When CuSO4 is dissolved in water, it partially disassociates into copper ions and sulfate ions as follows:
![]() (1)
(1)
If now a difference of potential is established between two copper electrodes in a copper sulfate solution, the Cu++ and SO42- ions begin to move in the resulting electric field. The doubly ionized copper ions move toward the negative plate, where they undergo the following reaction:
![]() (2)
(2)
The resultant neutral copper atom will (if the experiment is carefully conducted) remain deposited on the negative electrode. In the meantime, the SO42- ion attaches to a metallic copper atom on the positive electrode, as in the following reaction:
![]() (3)
(3)
This reaction provides the necessary free electrons to conserve charge in the circuit, and maintains the concentration of CuSO4 in the liquid solution.
Now, remembering that current is the rate of flow of charge,
![]() (4)
(4)
you should be able to calculate easily how many electrons have moved through the circuit in a given period if you measure the current in the circuit and the time interval during which the current flows. You are then able to determine how many copper atoms have been deposited, and by measuring the deposited mass, thus determine the mass of a single atom. Finally, since the gram-atomic mass (i.e., the mass of one mole of atoms) of elements is available in handbooks and other references, you can compute Avogadro's number.
EXPERIMENTAL ACTIVITY:
Clean the two copper strips at your work station carefully by rubbing them gently with steel wool, rinsing thoroughly with water, and drying with alcohol. When the strips are completely dry, weigh each one separately and record the masses to the nearest milligram (three decimal places). Be sure you are able to distinguish which strip had which mass. Have your partner weigh them a second time to independently check the masses. Accurate weighing is the key to good results in this experiment.
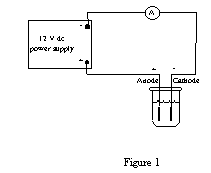
Construct the circuit shown in figure 1, but do not plug in the power supply until the instructor has checked your circuit. The lighter (less massive) of the two copper strips should be connected to the negative side of the power supply.
After the circuit is approved, plug in the power supply and turn it up quickly until the current is 400 mA. Maintain this current for 60 minutes. At the end of this time, turn off the power and carefully rinse, dry, and reweigh each of the strips.
Since you have weighed each strip before and after the exercise, you should be able to determine the mass change of each strip. Be sure to record the initial mass, the final mass, and the change in mass for each strip. Take the two values of mass change and average them. This average value of mass change is used in step 4 of the analysis. Be sure all values are neatly recorded in an organized data table.
ANALYSIS:
You should be able to make each of the following calculations with only a little mental effort. The first three calculations can be done while the experiment is in progress. Work independently of your partner in answering these questions. Your instructor will be happy to assist you over any rough spots. Show each calculation clearly and pay close attention to units and significant figures.
FINAL SUMMARY:
Report your value for Avogadro's number, and also your value of the mass of a single copper atom. Comment on your ability to measure such a small mass, and indicate any other conclusions that you are able to draw from this exercise.
ADDITIONAL QUESTIONS: